GCSE OCR Gateway Chemistry C3.1g–l Chemical Equations and the Mole

New OCR Gateway specification from September 2016 Higher tier: grades 9 to 4: 

In this and subsequent posts I’m simply going to explain and illustrate each learning objective as they come up in the topics in the new GCSE specification. 

I’m giving you my notes from each lesson.

You can really get ahead of your class if you follow this blog and all the posts that will appear here about the new GCSEs over the coming months.
 
This rejigging of the specification is just that: there is nothing really new here it has all been with us for the past half century at least. 

That written in italics is for the higher tier paper only.

C3 Chemical Reactions

C3.1 Introducing chemical reactions

Chemical Equations and the Mole

C3.1g recall and use the definitions of the Avogadro constant (in standard form) and of the mole and the calculation of the mass of one atom/molecule

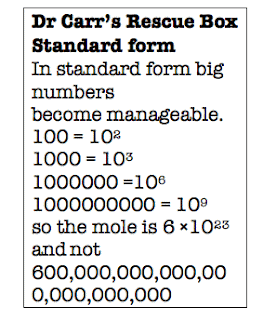
The Avogadro Constant is a number like the number in a dozen, 12 or in a score, 20 or in a gross, 144 or in a ream, 500.

A mole of particles or anything else is 6.02 × 10²³ in standard form.

Written out in full that’s 602,000,000,000,000,000,000,000.0

And a mole of atoms will have mass.

12.0000g of the isotope carbon C¹² contains exactly one mole of atoms and is the standard atomic mass.

The relative atomic mass of an element in units of grams contains exactly one mole of particles.

So 12.0000g carbon contains one mole of atoms.

23g of sodium metal contains one mole of sodium atoms.

But 32.00g of oxygen gas contains one mole of oxygen molecules O₂.

That’s because oxygen gas exists as diatomic molecules O₂ each with a RAM of 16.

So 124g of phosphorus contains one mole of phosphorus molecules P_4.   

That’s because phosphorus can exist as P4 molecules and each atom has a RAM of 31.

It works the same for molecules.

So 18g of water contains one mole of water molecules (H₂O) since the RAM of oxygen is 16 and that of hydrogen 1.0.

So two moles of water will contain 2 × 6 ×10²³ molecules.

And two moles will have a mass of 2 × 18g = 36g

C3.1h explain how the mass of a given substance is related to the amount of that substance in moles and vice versa

So from this we can say that

amt of substance (moles) × RMM(g/mol)  = mass of substance (g)

The relationship between mass of substance m (in grams), amount of substance n (in moles) and RAM or RMM M (in g/mol) is this:

                                          n   =     m/M

in words:

amount of substance n (moles)  =  mass of substance m (grams)

                                                                        RMM M (g/mol)

Problems:

1.            How many moles of chlorine in 71g?

2.            How many moles of neon in 40g?

3.            How many moles of sodium in 69g?

4.            How many moles of water in 72g?

5.            How many moles of ethanol (C₂H₅OH) in 92g?

6.            What mass is there in 6 moles of sulphur S₈?

7.            What mass is there in 10 moles of sodium carbonate (Na₂CO₃)?

8.            What mass is there in 1.5 moles of water?

9.            What mass is there in 4.67 moles of sugar (C₁₂H₂₂O₁₁)?

10.       What mass is there in 7.2 moles of sodium chloride (NaCl)?

Using the mole to calculate masses of reactants and products and to create balanced symbol equations.

C3.1k deduce the stoichiometry of an equation from the masses of reactants and products and explain the effect of a limiting quantity of a reactant.

Stoichiometry means the mole ratio of reacting substances in a chemical equation and the mole ratio of the products formed.

In this equation the stoichiometry is that two moles of hydrogen react with one mole of oxygen to form two moles of water.

2H₂(g)  +    O₂(g)    =    2H₂O(l)

If we know that 18g of aluminium reduce exactly 53.3g of iron(III)oxide to form 37.3g of iron and 34g of aluminium oxide then we can work out the stoichiometry of the equation.

Method:

1. Word equation	Aluminium +  iron oxide = iron  +  aluminium oxide
2. Masses (g)	18                   53.3               37.3               34
3. Molar masses (g/mol)	27                   160                56                   102
4. Moles (2./3.) i.e. use n=m/M	0.67               0.33               0.66               0.33
5. Simplest mole ratio	2                     1                     2                     1
6. Final equation	2Al    +      Fe2O3     =        2Fe       +        Al2O3

Now if we had used not 18g of aluminium but 30g there would not have been enough iron oxide to react with it. 

The iron (III) oxide is the limiting reactant. 

There would have been 12g of aluminium left over contaminating the iron formed.

So a limiting reactant means some reaction cannot take place because reactions have to follow the stoichiometry rules as in the example above where one mole of iron(III)oxide (no more no less) reacts exactly with two moles of aluminium.

The stoichiometry of a reaction states exactly how many moles of the two reactants will combine together. 

C3.1l use a balanced equation to calculate masses of reactants or products.

How to find the mass of product that forms in a reaction.

How much iron forms when carbon monoxide reduces 174g of iron(III)oxide in a Blast Furnace?

Method:

Word equation	Iron(III) oxide + carbon monoxide = iron   + carbon dioxide
Symbols	Fe2O3              +          3CO    =          2Fe     +          3CO2
Reacting masses (g)	160                            84                   112                132
Calculation
Answer	121.8g of iron

C3.1i recall and use the law of conservation of mass

The law of conservation of mass states that matter is neither created nor destroyed in a chemical change.

So the total mass of the reactants must be the same as the total mass of the products in a chemical change.

Example

2Al            +       Fe₂O₃         =       2Fe            +       Al₂O₃

54g                     160g          =       112g                    102g

total mass reactants 214g         total mass products 214g

This principle is true for every chemical change

C3.1j explain any observed changes in mass in non-enclosed systems during a chemical reaction and explain them using the particle model.

So when the mass of reactants does not equal the mass of product it is likely that a gas was given off

Example:

Mg(s)        +       2HCl(aq)            =       MgCl₂(aq)          +       H₂(g)

24g                     73g                       ≠       95g

mass of reactants                                 mass of observed products

The missing 2g is the hydrogen gas that escapes into the atmosphere.